Today is the day of FOMC, but I just realized this yesterday. Everyone knows that an FOMC probably shocks the market. However, I opened some directional positions on AMZN and INTC, seeing their earnings. I want to hedge out some of the market risk so I write this short passage to showcase how I do it and hoping somebody can educate me on this subject.
Positions I opened...
I was planning to reap two well-known effects from a credit put spread position.
Post-earnings announcement drift (PEAD):
It was discovered that the stock price not only moves at the instance when the earning is announced. The price will also drift slowly in the same direction in the following weeks or even months. For example, when there is a negative surprise in EPS, the price drops on the first day. The price will continuously drop further. Behavioral finance guys said this is because of the slow reaction of stupid investors.
Variance risk premium (VRP):
The option prices are usually overpriced. The market has an overestimation of volatility, higher than the realized one. Explaining with a Black-Scholes model, it means that usually, the overestimated vol input will also produce an overestimated price output. Therefore, comparing the implied volatility and my own estimation of the realized volatility, there is a premium I can collect from selling options. Of course, there is a risk for this risk premium (no shit...). The realized vol may actually be extremely high.
Thus, I opened two positive delta credit put spread on AMZN and INTC, seeing their positive earning surprises.
Hedging the market
Unfortunately, the FOMC will probably shock the market, either up or down. This is a binary event that I don't have the knowledge to forecast. I'm not going to speculate on this event too, but the bad news is that AMZN and INTC may be affected too. If the market drops, AMZN and INTC will probably drop too. My positive delta positions are gonna lose.
Therefore, I want to hedge this market risk. I definitely don't want to be delta-neutral on AMZN and INTC, so I need to find an asset that (1) moving with the market, (2) not very correlated with AMZN and INTC, and (3) it better be soaring. Hence, I can have a negative delta position on this asset, so that when the market is down, I hope this asset will go down too and hedge out my loss on AMZN and INTC.
I found that F just had a negative surprise on its earnings. Again, I opened a short position but with calls this time so it's a credit call spread. Thus, I am hoping it (1) drops with the market, (2) it drops individually.
Overall Positions
| AMZN | INTC | F | |
| Delta | (+) Long | (+) Long | (-) Short |
| Gamma | (-) Short | (-) Short | (-) Short |
| Theta | (+) Long | (+) Long | (+) Long |
| Vega | (-) Short | (-) Short | (-) Short |
Basically, except for the delta exposure, others are identically for every stock, consistent with my intention of shorting vol.
Choosing exposures of the hedge
My plan is to calculate the beta for every stock. Then, estimate the delta realized on my portfolio when the market moves by 1% (the stocks will move by (1 * beta)%). Based on this market delta, I choose the delta exposure on F to offset the portfolio's market delta. The logic behind is akin to a long-short portfolio.
Here is my position, but I miscalculated when I opened the position on F:
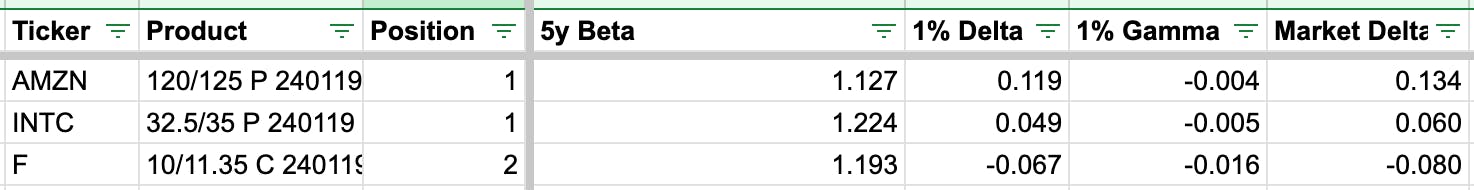
So 1% delta is estimated by:
$$1\%Delta=\#\ of \ Contracts\times(Spot\times1\%\times\Delta+\frac{(Spot\times1\%)(Spot\times1\%+1)}{2}\times\Gamma)$$
The beta is estimated by a linear regression of the stock return (%) on market return (%). Thus, the market delta is simply scaling the 1% delta by the beta:
$$MarketDelta = 1\%Delta\times\beta$$
Therefore, my overall delta exposure to the market is:
$$TotalMarketDelta=0.134+0.06=0.194$$
We need -19.4 market delta on F. Solving for the normal delta:
$$1\%Delta = MarketDelta\div\beta=-0.194\div1.193=-0.10$$
I ignored gamma for simplicity:
$$\#\ of\ Contracts\times\Delta= \frac{1\%Delta}{Spot\times1\%}=\frac{-0.10}{9.82\times1\%}=-1.02$$
By my estimation, I need around a -100 delta position on F to fully hedge out market risk but I only opened two -30 delta spreads (-60 delta in total).
But gladly I miscalculated it, as I just saw a bit up on F's price. I'm also unsure about the effectiveness of this hedging method. Maybe I'm just overtrading and paying mortgages for market makers.
Some possible flaws
To my knowledge, I know there are some risks.
Estimation error on beta:
Beta is usually unstable. Everyone knows that CAPM is only an analytical model without much explanatory power empirically. Issues like OLS, evolving market, and idiosyncratic risk, can all be contributing to this error. I simply used the last 5 years' daily return to estimate it, hoping the market wouldn't work against me. 🙏🙏🙏
Convexity of delta (gamma):
I didn't include the gamma in the calculations to make my life easier. When the market becomes crazy and maybe soars 10%, the gammas will cause an imbalance of deltas. It should be very unlikely, god knows!
